DALDØS VS KALAH

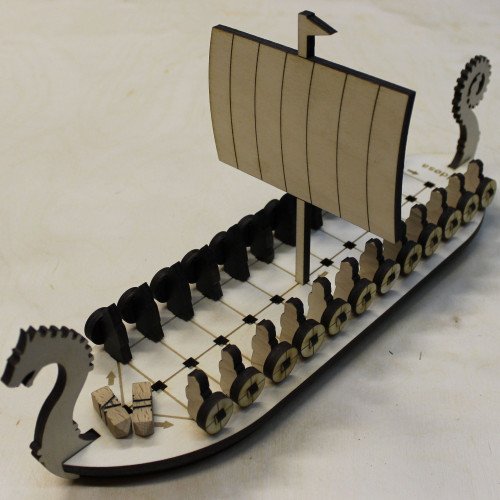
Daldøs is a running-fight board game only known from a few coastal locations in southern Scandinavia, where its history can be traced back to around 1800. The game is notable for its unusual four-sided dice (stick or long dice). In Denmark it is known as daldøs in Northern and Western Jutland (Mors, Thisted and Fanø), and possibly as daldos on Bornholm. In Norway it is known under the name of daldøsa from Jæren, where, unlike in Denmark, a continuous tradition of the daldøs game exists. Daldøs has much in common with some games in the sáhkku family of Sámi board games. Sáhkku is known to have been played among Sámi on the northern coast and eastern-central inland of Sápmi, far away from Jæren and Denmark. Otherwise, the closest relatives of this game appear to be the tâb games from Northern Africa and South-western Asia, possibly apart from one unlabelled diagram in a codex from Southern England. The board is boat-shaped and has three parallel rows of holes, two of which (A and B) have 16 holes each, while the middle row has an extra hole in the prow of the ship. Each player has 16 spatula-shaped pieces with a bottom end fitting into the holes of the board. One player has pieces that are rather wide and thin; whereas the other player's pieces are more obelisk-shaped. At the beginning of the game, player A's pieces are placed in the holes of row A so that the spatulas are perpendicular to the row (un-dalled), and equivalently for player B. Later in the game, the pieces will be turned (fordallede, or dalled) so that the spatula is parallel to the rows. Two special dice are used. Each die is a four-sided long die with pyramidal or rounded ends, preventing the die from standing on end. They may be about 2 by 2 cm in cross section, and 4 cm long. The four sides are marked A (with the value 1, called dallen, i.e. the dal), II (2, probably called døs), III (3) and IIII (4). According to some sources, the dal is opposite to III.

See statistics

Kalah, also called Kalaha or Mancala, is a game in the mancala family invented in the United States by William Julius Champion, Jr. in 1940. This game is sometimes also called "Kalahari", possibly by false etymology from the Kalahari desert in Namibia. As the most popular and commercially available variant of mancala in the West, Kalah is also sometimes referred to as Warri or Awari, although those names more properly refer to the game Oware. For most of its variations, Kalah is a solved game with a first-player win if both players play perfect games. The Pie rule can be used to balance the first-player's advantage. Mark Rawlings has written a computer program to extensively analyze both the "standard" version of Kalah and the "empty capture" version, which is the primary variant. The analysis was made possible by the creation of the largest endgame databases ever made for Kalah. They include the perfect play result of all 38,902,940,896 positions with 34 or fewer seeds. In 2015, for the first time ever, each of the initial moves for the standard version of Kalah(6,4) and Kalah(6,5) have been quantified: Kalah(6,4) is a proven win by 8 for the first player and Kalah(6,5) is a proven win by 10 for the first player. In addition, Kalah(6,6) with the standard rules has been proven to be at least a win by 4. Further analysis of Kalah(6,6) with the standard rules is ongoing. For the "empty capture" version, Geoffrey Irving and Jeroen Donkers (2000) proved that Kalah(6,4) is a win by 10 for the first player with perfect play, and Kalah(6,5) is a win by 12 for the first player with perfect play. Anders Carstensen (2011) proved that Kalah(6,6) was a win for the first player. Mark Rawlings (2015) has extended these "empty capture" results by fully quantifying the initial moves for Kalah(6,4), Kalah(6,5), and Kalah(6,6). With searches totaling 106 days and over 55 trillion nodes, he has proven that Kalah(6,6) is a win by 2 for the first player with perfect play. This was a surprising result, given that the "4-seed" and "5-seed" variations are wins by 10 and 12, respectively. Kalah(6,6) is extremely deep and complex when compared to the 4-seed and 5-seed variations, which can now be solved in a fraction of a second and less than a minute, respectively.

See statistics